discrete logarithm

Basic definition

When we understand discrete logarithms, let's first look at a few basic definitions.

Definition 1

In group G, g is the generator of G, that is, each element in group G can be written as $y=g^k$, which we call k is the logarithm of y in group G.

Definition 2

Let $m\geq 1$, $(a,m)=1$, let $a^d \equiv 1\pmod m$ be the smallest positive integer d called a to the exponent or order of modulo m, we will generally It is recorded as $\delta_m(a)$.

Definition 3

When $\delta_m(a)=\varphi(m)$, a is called the original root of modulo m, which is called the original root of m.

Some properties

Nature 1

The smallest positive integer $d$ that makes $a^d \equiv 1\pmod m$ true must have $d\mid\varphi(m)$.

Property 2

The necessary and sufficient condition for the existence of the original root of the modulo $m$ is $m=2,4,p^{\alpha}, 2p^{\alpha}$ , where $p$ is an odd prime, $\alpha$ is positive Integer.

Discrete logarithm problem

Knowing $g,p,y$, for the equation $y\equiv g^x \pmod p$ , solving $x$ is a difficult problem. But when $p$ has certain characteristics, it can be solved. For example, the order of this group is a smooth number.

It is this problem that constitutes a large part of modern cryptography, including Diffie–Hellman key exchange, ElGamal algorithm, ECC, etc.

Discrete logarithm solution

暴暴

Given $y\equiv g^x \pmod p$, we can violently enumerate $x$ to get the true value of $x$.

Baby-step giant-step

This method is often referred to as a small step, which uses the idea of an intermediate encounter attack.

We can make $x=im+j$, where $m= \lceil \sqrt n\rceil$ , then the integers i and j are all in the range 0 to m.

therefore

$$y = g ^ x = g ^ {im + j}$$

That is

$$y(g^{-m})^i=g^j$$

Then we can enumerate all the j and calculate it and store it in a set S, then we enumerate i again, calculate $y(g^{-m})^i$, once we find the calculation The result in the set S shows that we got a collision and got i and j.

This is obviously a way of compromise between time and space. We convert a $O(n)$ time complexity, $O(1)$ space complexity algorithm to a $O(\sqrt n)$ The algorithm of time complexity and space complexity of $O(\sqrt n)$.

among them

• Each increment of j means "baby-step", multiplied by $g$ at a time.
• Each increment of i means "giant-step", multiplied by $g^{-m}$ at a time.

def bsgs(g, y, p):

    m = int(ceil(sqrt(p - 1)))

    S = {pow(g, j, p): j for j in range(m)}

    gs = pow(g, p - 1 - m, p)

    for i in range(m):

        if y in S:

            return i * m + S[y]

y = y * gs% p
    return None

Pollard's ρ algorithm

We can solve the above problem with the time complexity of $O(\sqrt n)$ and the space complexity of $O(1)$. Please use Google for your specific principles.

Pollard’s kangaroo algorithm

If we know that the range of x is $a \leq x \leq b$, then we can solve the above problem with the time complexity of $O(\sqrt{ba})$. Please use Google for your specific principles.

Pohlig-Hellman algorithm

Let's assume that the above mentioned group has a rank of $n$ for the element $g$, and $n$ is a smooth number: $n=\prod\limits_{i=1}^r p_i^{e_i}$.

1. For each $i \in \{1,\ldots,r\}$ :
2. Calculate $g_i \equiv g^{n/p_i^{e_i}} \pmod m$. According to the Lagrange theorem, the order of $g_i$ in the group is $p_i^{e_i}$.
3. Calculate $y_i \equiv y^{n/p_i^{e_i}} \equiv g^{xn/p_i^{e_i}} \equiv g_i^{x} \equiv g_i^{x \bmod p_i^{e_i }} \equiv g_i^{x_i} \pmod m$, here we know $y_i,m,g_i$, and $x_i$ ranges from $[0,p_i^{e_i})$, by $n$ is a Smooth numbers, the range is small, so we can quickly find $x_i$ using methods such as Pollard's kangaroo algorithm.
4. According to the above derivation, we can get for $i \in \{1,\ldots,r\}$ , $x \equiv x_i \pmod{p_i^{e_i}}$ , which can be solved by Chinese remainder theorem .

The above process can be briefly described in the following figure:

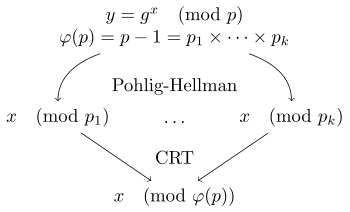
The complexity is $O\left(\sum\limits _i e_i\left(\log n+\sqrt{p_i}\right)\right)$, and it can be seen that the complexity is still very low.

But when $n$ is prime, $m=2n+1$, then the complexity and $O(\sqrt m)$ are almost indistinguishable.

2018 国赛crackme java

code show as below

import java.math.BigInteger;

import java.util.Random;


public class Test1 {

    static BigInteger two =new BigInteger("2");

    static BigInteger p = new BigInteger("11360738295177002998495384057893129964980131806509572927886675899422214174408333932150813939357279703161556767193621832795605708456628733877084015367497711");

    static BigInteger h= new BigInteger("7854998893567208831270627233155763658947405610938106998083991389307363085837028364154809577816577515021560985491707606165788274218742692875308216243966916");



    /*

     Alice write the below algorithm for encryption.

     The public key {p, h} is broadcasted to everyone.

    @param val: The plaintext to encrypt.

        We suppose val only contains lowercase letter {a-z} and numeric charactors, and is at most 256 charactors in length.

    */

    public static String pkEnc(String val){

        BigInteger[] ret = new BigInteger[2];

        BigInteger bVal=new BigInteger(val.toLowerCase(),36);

        BigInteger r =new BigInteger(new Random().nextInt()+"");

ret [0] = two.modPow (r, p);entitled [1] = h.modPow (r, p) .multiply (bVal);
return right [0] .toString (36) + "==" + ret [1] .toString (36);
    }



    /* Alice write the below algorithm for decryption. x is her private key, which she will never let you know.

    public static String skDec(String val,BigInteger x){

        if(!val.contains("==")){

            return null;

        }

        else {

            BigInteger val0=new BigInteger(val.split("==")[0],36);

            BigInteger val1=new BigInteger(val.split("==")[1],36);

            BigInteger s=val0.modPow(x,p).modInverse(p);

            return val1.multiply(s).mod(p).toString(36);

        }

    }

   */



    public static void main(String[] args) throws Exception {

        System.out.println("You intercepted the following message, which is sent from Bob to Alice:");

        BigInteger bVal1=new BigInteger("a9hgrei38ez78hl2kkd6nvookaodyidgti7d9mbvctx3jjniezhlxs1b1xz9m0dzcexwiyhi4nhvazhhj8dwb91e7lbbxa4ieco",36);

    BigInteger bVal2=new BigInteger("2q17m8ajs7509yl9iy39g4znf08bw3b33vibipaa1xt5b8lcmgmk6i5w4830yd3fdqfbqaf82386z5odwssyo3t93y91xqd5jb0zbgvkb00fcmo53sa8eblgw6vahl80ykxeylpr4bpv32p7flvhdtwl4cxqzc",36);

    BigInteger r =new BigInteger(new Random().nextInt()+"");

    System.out.println(r);

        System.out.println(bVal1);

    System.out.println(bVal2);

    System.out.println("a9hgrei38ez78hl2kkd6nvookaodyidgti7d9mbvctx3jjniezhlxs1b1xz9m0dzcexwiyhi4nhvazhhj8dwb91e7lbbxa4ieco==2q17m8ajs7509yl9iy39g4znf08bw3b33vibipaa1xt5b8lcmgmk6i5w4830yd3fdqfbqaf82386z5odwssyo3t93y91xqd5jb0zbgvkb00fcmo53sa8eblgw6vahl80ykxeylpr4bpv32p7flvhdtwl4cxqzc");

        System.out.println("Please figure out the plaintext!");

    }

}

Basic function is calculation

$ R_0 = 2 ^ r way $ p

$ R_1 = b * h ^ r way $ p

It can be found that the range of r is $[0,2^{32})$, so we can use the BSGS algorithm as follows

from sage.all import *



c1 = int(

    'a9hgrei38ez78hl2kkd6nvookaodyidgti7d9mbvctx3jjniezhlxs1b1xz9m0dzcexwiyhi4nhvazhhj8dwb91e7lbbxa4ieco',

    36

)

c2 = int(

    '2q17m8ajs7509yl9iy39g4znf08bw3b33vibipaa1xt5b8lcmgmk6i5w4830yd3fdqfbqaf82386z5odwssyo3t93y91xqd5jb0zbgvkb00fcmo53sa8eblgw6vahl80ykxeylpr4bpv32p7flvhdtwl4cxqzc',

    36

)

print c1, c2

p = 11360738295177002998495384057893129964980131806509572927886675899422214174408333932150813939357279703161556767193621832795605708456628733877084015367497711

h = 7854998893567208831270627233155763658947405610938106998083991389307363085837028364154809577816577515021560985491707606165788274218742692875308216243966916

# generate the group

const2 = 2
const2 = Mod (const2, p)
c1 = Mod(c1, p)

c2 = Mod(c2, p)

h = Mod(h, p)

print '2', bsgs(const2, c1, bounds=(1, 2 ^ 32))



r = 152351913



num = long(c2 / (h**r))

Surely Print

Reference

• Elementary number theory, Pan Chengdong, Pan Chengyu

• https://ee.stanford.edu/~hellman/publications/28.pdf

• https://en.wikipedia.org/wiki/Pohlig%E2%80%93Hellman_algorithm#cite_note-Menezes97p108-2

• https://fortenf.org/e/crypto/2017/12/03/survey-of-discrete-log-algos.html

---

本页面的全部内容在 CC BY-NC-SA 4.0 协议之条款下提供，附加条款亦可能应用。

Comments